Po-Shen Loh
Professor | Carnegie Mellon University
Pittsburgh, PA, UNITED STATES
Po-Shen Loh is a social entrepreneur, working across the spectrum of mathematics, education and health care.
Biography
Po-Shen Loh is a social entrepreneur, working across the spectrum of mathematics, education and health care, all around the world. He is a math professor at Carnegie Mellon University and the national coach of the USA International Mathematical Olympiad team. He has pioneered innovations ranging from a scalable way to learn math live online at comparable engagement to live-streaming platforms, to a new way to control pandemics, such as COVID-19, by leveraging self-interest. His scientific research considers a variety of questions that lie at the intersection of combinatorics (the study of discrete systems), probability theory and computer science.
Areas of Expertise (5)
Combinatorics
Mathematics
Math Education
Probability Theory
Computer Science
Media Appearances (6)
Why this math professor is putting actors in classrooms
CNN online
2024-03-18
Po-Shen Loh is a man on a mission. A professor of mathematics at Carnegie Mellon university, in Pennsylvania, he believes that reimagining the way we teach can help future-proof youngsters in a world where AI poses a growing threat to job security.
AI will spur entrepreneurialism, make everyone a 'mini-boss': Po-Shen Loh
Fox Business tv
2023-06-08
Carnegie Mellon University professor Po-Shen Loh tells 'The Big Money Show' how he teaches kids to outsmart A.I.
America’s Math Coach Is Teaching Fifth-Graders to Outsmart AI
The Wall Street Journal online
2023-05-25
Teaching math the Po-Shen Loh way meant rethinking the way math is taught. The precocious middle-schoolers in his program take extracurricular math classes from exceptional high-schoolers, but there’s another person lurking in the virtual classrooms: a drama coach. Loh pays comedians, actors and theater majors to provide real-time feedback on the teachers’ performance, with the aim of making combinatorics as entertaining as YouTube, Twitch and whatever the students were escaping Zoom to watch.
This CMU professor is making math classes less dull. Meet Live
Technical.ly online
2023-01-25
Po-Shen Loh knows there are limits to Zoom instruction, so to make math classes more engaging, he created an interactive catalog of courses taught by some of the best math students in the country.
Contact Tracing Didn't Defeat Covid. Here's How It Could | Opinion
Bloomberg online
2022-01-28
Remember contact tracing? Early in the Covid-19 pandemic, it was seen as the first line of defense against the virus. The idea was that public health workers could be mobilized to contact everyone who’d been infected or exposed, and then would warn friends, family, neighbors and colleagues to lie low.
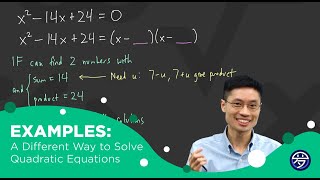
Mathematician Finds Easier Way to Solve Quadratic Equations
Popular Mechanics online
2020-12-08
Quadratic equations are polynomials that include an x², and teachers use them to teach students to find two solutions at once. The new process, developed by Dr. Po-Shen Loh at Carnegie Mellon University, goes around traditional methods like completing the square and turns finding roots into a simpler thing involving fewer steps that are also more intuitive.
Media
Publications:
Documents:
Photos:
Videos:
Audio/Podcasts:
Industry Expertise (3)
Computer Software
Education/Learning
Health Care - Services
Accomplishments (3)
William H. and Frances S. Ryan Award (professional)
2019
NSF CAREER Award (professional)
n/a
Presidential Early Career Award for Scientists and Engineers (professional)
2019
Education (3)
Princeton University: Ph.D., Mathematics 2010
California Institute of Technology: B.S., Mathematics 2004
Cambridge University: M.S, Mathematics 2005
Links (5)
Event Appearances (1)
Commencement Keynote,,The Future of Education
(2018) Duquesne University School of Education
Articles (2)
The random k-matching-free process
Random Structures and Algorithms 53(4)2017 Let $\mathcal{P}$ be a graph property which is preserved by removal of edges, and consider the random graph process that starts with the empty $n$-vertex graph and then adds edges one-by-one, each chosen uniformly at random subject to the constraint that $\mathcal{P}$ is not violated. These types of random processes have been the subject of extensive research over the last 20 years, having striking applications in extremal combinatorics, and leading to the discovery of important probabilistic tools. In this paper we consider the $k$-matching-free process, where $\mathcal{P}$ is the property of not containing a matching of size $k$.
Distance-Uniform Graphs with Large Diameter
SIAM Journal on Discrete Mathematics2019 An ϵ-distance-uniform graph is one in which from every vertex, all but an ϵ-fraction of the remaining vertices are at some fixed distance d, called the critical distance. We consider the maximum possible value of d in an ϵ-distance-uniform graph with n vertices. We show that for 1n≤ϵ≤1logn, there exist ϵ-distance-uniform graphs with critical distance 2Ω(lognlogϵ−1), disproving a conjecture of Alon et al. that d can be at most logarithmic in n. We also show that our construction is best possible, in the sense that an upper bound on d of the form 2O(lognlogϵ−1) holds for all ϵ and n.
Social